MINLPLib
A Library of Mixed-Integer and Continuous Nonlinear Programming Instances
Home // Instances // Documentation // Download // Statistics
Instance waterx
| Formatsⓘ | ams gms mod nl osil py |
| Primal Bounds (infeas ≤ 1e-08)ⓘ | |
| Other points (infeas > 1e-08)ⓘ | |
| Dual Boundsⓘ | 807.00733870 (BARON) 612.91897410 (COUENNE) 723.22038680 (GUROBI) 909.02786260 (LINDO) 908.89506120 (SCIP) 0.00000000 (SHOT) 146.87770900 (XPRESS) |
| Referencesⓘ | Brooke, Anthony, Drud, Arne S, and Meeraus, Alexander, Modeling Systems and Nonlinear Programming in a Research Environment. In Ragavan, R and Rohde, S M, Eds, Computers in Engineering, Vol. III, ACME, 1985. Drud, Arne S and Rosenborg, A, Dimensioning Water Distribution Networks, Masters thesis, Institute of Mathematical Statistics and Operations Research, Technical University of Denmark, 1973. In Danish. |
| Sourceⓘ | GAMS Model Library model waterx |
| Applicationⓘ | Water Network Design |
| Added to libraryⓘ | 01 May 2001 |
| Problem typeⓘ | MBNLP |
| #Variablesⓘ | 70 |
| #Binary Variablesⓘ | 14 |
| #Integer Variablesⓘ | 0 |
| #Nonlinear Variablesⓘ | 46 |
| #Nonlinear Binary Variablesⓘ | 0 |
| #Nonlinear Integer Variablesⓘ | 0 |
| Objective Senseⓘ | min |
| Objective typeⓘ | linear |
| Objective curvatureⓘ | linear |
| #Nonzeros in Objectiveⓘ | 4 |
| #Nonlinear Nonzeros in Objectiveⓘ | 0 |
| #Constraintsⓘ | 54 |
| #Linear Constraintsⓘ | 38 |
| #Quadratic Constraintsⓘ | 1 |
| #Polynomial Constraintsⓘ | 0 |
| #Signomial Constraintsⓘ | 15 |
| #General Nonlinear Constraintsⓘ | 0 |
| Operands in Gen. Nonlin. Functionsⓘ | |
| Constraints curvatureⓘ | indefinite |
| #Nonzeros in Jacobianⓘ | 236 |
| #Nonlinear Nonzeros in Jacobianⓘ | 60 |
| #Nonzeros in (Upper-Left) Hessian of Lagrangianⓘ | 130 |
| #Nonzeros in Diagonal of Hessian of Lagrangianⓘ | 42 |
| #Blocks in Hessian of Lagrangianⓘ | 16 |
| Minimal blocksize in Hessian of Lagrangianⓘ | 2 |
| Maximal blocksize in Hessian of Lagrangianⓘ | 3 |
| Average blocksize in Hessian of Lagrangianⓘ | 2.875 |
| #Semicontinuitiesⓘ | 0 |
| #Nonlinear Semicontinuitiesⓘ | 0 |
| #SOS type 1ⓘ | 0 |
| #SOS type 2ⓘ | 0 |
| Minimal coefficientⓘ | 6.9000e-02 |
| Maximal coefficientⓘ | 2.5020e+03 |
| Infeasibility of initial pointⓘ | 723.6 |
| Sparsity Jacobianⓘ | 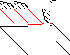 |
| Sparsity Hessian of Lagrangianⓘ |  |
$offlisting
*
* Equation counts
* Total E G L N X C B
* 55 27 0 28 0 0 0 0
*
* Variable counts
* x b i s1s s2s sc si
* Total cont binary integer sos1 sos2 scont sint
* 71 57 14 0 0 0 0 0
* FX 0
*
* Nonzero counts
* Total const NL DLL
* 241 181 60 0
*
* Solve m using MINLP minimizing objvar;
Variables x1,x2,x3,x4,x5,x6,x7,x8,x9,x10,x11,x12,x13,x14,x15,x16,x17,x18,x19
,x20,x21,x22,x23,x24,x25,x26,x27,x28,x29,x30,x31,x32,x33,x34,x35,x36
,x37,x38,x39,x40,x41,x42,x43,x44,x45,x46,x47,x48,x49,x50,x51,x52,x53
,x54,x55,objvar,x57,b58,b59,b60,b61,b62,b63,b64,b65,b66,b67,b68,b69
,b70,b71;
Positive Variables x1,x2,x3,x4,x5,x6,x7,x8,x9,x10,x11,x12,x13,x14,x15,x16,x17
,x18,x19,x20,x21,x22,x23,x24,x25,x26,x27,x28,x51,x52;
Binary Variables b58,b59,b60,b61,b62,b63,b64,b65,b66,b67,b68,b69,b70,b71;
Equations e1,e2,e3,e4,e5,e6,e7,e8,e9,e10,e11,e12,e13,e14,e15,e16,e17,e18,e19
,e20,e21,e22,e23,e24,e25,e26,e27,e28,e29,e30,e31,e32,e33,e34,e35,e36
,e37,e38,e39,e40,e41,e42,e43,e44,e45,e46,e47,e48,e49,e50,e51,e52,e53
,e54,e55;
e1.. - x1 - x2 - x3 + x15 + x16 + x17 + x51 =E= 0;
e2.. - x4 - x5 - x6 - x7 + x18 + x19 + x20 + x21 + x52 =E= 0;
e3.. x1 + x4 - x8 - x9 - x10 - x11 - x15 - x18 + x22 + x23 + x24 + x25
=E= 1.212;
e4.. x2 + x8 + x12 - x16 - x22 - x26 =E= 0.452;
e5.. x9 - x12 + x13 - x23 + x26 - x27 =E= 0.245;
e6.. x5 + x10 - x13 - x14 - x19 - x24 + x27 + x28 =E= 0.652;
e7.. x6 + x14 - x20 - x28 =E= 0.252;
e8.. x3 + x7 + x11 - x17 - x21 - x25 =E= 0.456;
e9.. -(1.5722267648148*x1 + 1.5722267648148*x15)*(x1 - x15)/x29**5.33 + x43
- x45 =E= 0;
e10.. -(1.32004857865156*x2 + 1.32004857865156*x16)*(x2 - x16)/x30**5.33 + x43
- x46 =E= 0;
e11.. -(2.57705917665854*x3 + 2.57705917665854*x17)*(x3 - x17)/x31**5.33 + x43
- x50 =E= 0;
e12.. -(2.06257339263358*x4 + 2.06257339263358*x18)*(x4 - x18)/x32**5.33 + x44
- x45 =E= 0;
e13.. -(2.40235218067626*x5 + 2.40235218067626*x19)*(x5 - x19)/x33**5.33 + x44
- x48 =E= 0;
e14.. -(1.339*x6 + 1.339*x20)*(x6 - x20)/x34**5.33 + x44 - x49 =E= 0;
e15.. -(1.37419139860501*x7 + 1.37419139860501*x21)*(x7 - x21)/x35**5.33 + x44
- x50 =E= 0;
e16.. -(1.2916134290104*x8 + 1.2916134290104*x22)*(x8 - x22)/x36**5.33 + x45
- x46 =E= 0;
e17.. -(1.60230396616872*x9 + 1.60230396616872*x23)*(x9 - x23)/x37**5.33 + x45
- x47 =E= 0;
e18.. -(1.339*x10 + 1.339*x24)*(x10 - x24)/x38**5.33 + x45 - x48 =E= 0;
e19.. -(2.14329116080854*x11 + 2.14329116080854*x25)*(x11 - x25)/x39**5.33
+ x45 - x50 =E= 0;
e20.. -(1.24561882211213*x12 + 1.24561882211213*x26)*(x12 - x26)/x40**5.33
- x46 + x47 =E= 0;
e21.. -(1.15157500841239*x13 + 1.15157500841239*x27)*(x13 - x27)/x41**5.33
- x47 + x48 =E= 0;
e22.. -(2.06257339263358*x14 + 2.06257339263358*x28)*(x14 - x28)/x42**5.33
+ x48 - x49 =E= 0;
e23.. -(1.02*x51*(-6.5 + x43) + 1.02*x52*(-3.25 + x44)) + x53 =E= 0;
e24.. -0.069*(1526.43375224737*x29**1.29 + 1281.60056179763*x30**1.29 +
2501.99920063936*x31**1.29 + 2002.49843945008*x32**1.29 +
2332.38075793812*x33**1.29 + 1300*x34**1.29 + 1334.16640641263*x35**1.29
+ 1253.99362039845*x36**1.29 + 1555.6349186104*x37**1.29 + 1300*x38**
1.29 + 2080.86520466848*x39**1.29 + 1209.33866224478*x40**1.29 +
1118.03398874989*x41**1.29 + 2002.49843945008*x42**1.29) + x54 =E= 0;
e25.. - 0.2*x51 - 0.17*x52 + x55 =E= 0;
e26.. - 10*x53 - x54 - 10*x55 + objvar - x57 =E= 0;
e27.. - x1 - x2 - x3 - x4 - x5 - x6 - x7 - x8 - x9 - x10 - x11 - x12 - x13
- x14 - x15 - x16 - x17 - x18 - x19 - x20 - x21 - x22 - x23 - x24 - x25
- x26 - x27 - x28 + x57 =E= 0;
e28.. x1 - 2*b58 =L= 0;
e29.. x2 - 2*b59 =L= 0;
e30.. x3 - 2*b60 =L= 0;
e31.. x4 - 2*b61 =L= 0;
e32.. x5 - 2*b62 =L= 0;
e33.. x6 - 2*b63 =L= 0;
e34.. x7 - 2*b64 =L= 0;
e35.. x8 - 2*b65 =L= 0;
e36.. x9 - 2*b66 =L= 0;
e37.. x10 - 2*b67 =L= 0;
e38.. x11 - 2*b68 =L= 0;
e39.. x12 - 2*b69 =L= 0;
e40.. x13 - 2*b70 =L= 0;
e41.. x14 - 2*b71 =L= 0;
e42.. x15 + 2*b58 =L= 2;
e43.. x16 + 2*b59 =L= 2;
e44.. x17 + 2*b60 =L= 2;
e45.. x18 + 2*b61 =L= 2;
e46.. x19 + 2*b62 =L= 2;
e47.. x20 + 2*b63 =L= 2;
e48.. x21 + 2*b64 =L= 2;
e49.. x22 + 2*b65 =L= 2;
e50.. x23 + 2*b66 =L= 2;
e51.. x24 + 2*b67 =L= 2;
e52.. x25 + 2*b68 =L= 2;
e53.. x26 + 2*b69 =L= 2;
e54.. x27 + 2*b70 =L= 2;
e55.. x28 + 2*b71 =L= 2;
* set non-default bounds
x29.lo = 0.15; x29.up = 2;
x30.lo = 0.15; x30.up = 2;
x31.lo = 0.15; x31.up = 2;
x32.lo = 0.15; x32.up = 2;
x33.lo = 0.15; x33.up = 2;
x34.lo = 0.15; x34.up = 2;
x35.lo = 0.15; x35.up = 2;
x36.lo = 0.15; x36.up = 2;
x37.lo = 0.15; x37.up = 2;
x38.lo = 0.15; x38.up = 2;
x39.lo = 0.15; x39.up = 2;
x40.lo = 0.15; x40.up = 2;
x41.lo = 0.15; x41.up = 2;
x42.lo = 0.15; x42.up = 2;
x43.lo = 6.5;
x44.lo = 3.25;
x45.lo = 16.58;
x46.lo = 14.92;
x47.lo = 12.925;
x48.lo = 12.26;
x49.lo = 8.76;
x50.lo = 16.08;
x51.up = 2.5;
x52.up = 6;
* set non-default levels
x29.l = 0.547722557505166;
x30.l = 0.547722557505166;
x31.l = 0.547722557505166;
x32.l = 0.547722557505166;
x33.l = 0.547722557505166;
x34.l = 0.547722557505166;
x35.l = 0.547722557505166;
x36.l = 0.547722557505166;
x37.l = 0.547722557505166;
x38.l = 0.547722557505166;
x39.l = 0.547722557505166;
x40.l = 0.547722557505166;
x41.l = 0.547722557505166;
x42.l = 0.547722557505166;
x43.l = 11.5;
x44.l = 8.25;
x45.l = 21.58;
x46.l = 19.92;
x47.l = 17.925;
x48.l = 17.26;
x49.l = 13.76;
x50.l = 21.08;
x51.l = 0.961470588235294;
x52.l = 2.30752941176471;
Model m / all /;
m.limrow=0; m.limcol=0;
m.tolproj=0.0;
$if NOT '%gams.u1%' == '' $include '%gams.u1%'
$if not set MINLP $set MINLP MINLP
Solve m using %MINLP% minimizing objvar;
Last updated: 2025-08-07 Git hash: e62cedfc